Monty & the Doors

A couple of days back I was reading an article about statistical method in experiments in primate behaviour and the writer mentioned The Monty Hall Problem as a possible source of unintentional introduced error or experimenter bias.

Now The Monty Hall Problem is a fascinating mathematical conundrum, and since I know those kinds of things are always of interest to Cow Readers, I thought those of you who are not familiar with this puzzle might like to exercise your mental muscles on it.

The Monty Hall Problem goes like this:

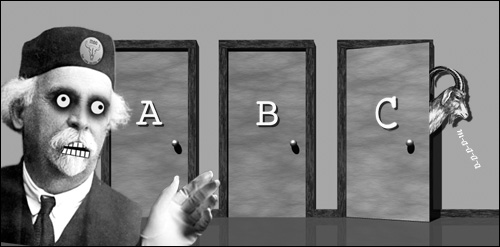
You are on a game show with your host Monty Hall who is offering you the chance to walk away with the Car of Your Dreams. He shows you three doors, A, B & C.

“The Car of Your Dreams is behind one of these doors,” he says, “The other two doors each conceal a goat. As your Games Master, only I know which door conceals which object. Now, please choose a door to claim your prize!’

You choose your door. You tell Monty “I have chosen Door B!”

“Well done!” he says. “I knew you were a contestant of superior ability! But before we open your door, I’m going to open one of the other doors and show you what’s behind it.” He opens Door C to reveal a goat. “Now that you’ve seen what’s behind Door C,” he says, “I’m going to give you a special opportunity to stick with your chosen door, Door B, or change your choice to the other remaining door, Door A. I’ll give you ten seconds to have a think about it!”

Here’s the question: To win the car, is there any advantage in changing your mind and swapping from your initial choice of Door B to Door A?

Answers on my desk by end of class.