Tetherd Cow Ahead

Well, enough of all that statistical probability jiggery-pokery. Instead, go read this article about which I ask, ‘What do they mean about black cats being bad luck?’

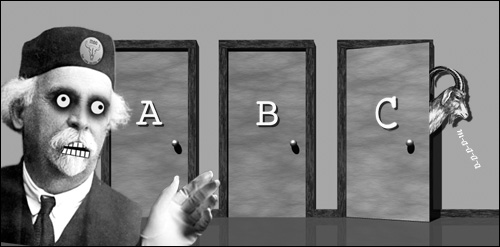
The correct answer to The Monty Hall Problem is: Yes, you should definitely change your choice when Monty gives you the opportunity. You will improve your odds of walking away with the car from your initial 33.3% to an impressive 66.6%.

Those of you who said that your chances remain the same as they were to start with, or improve to an even 50/50, are in error. I know, it seems bizarre on the face of it: if you change your mind, your chances of winning the car are not just better, but substantially better. But how can that possibly be?

I think the best way to approach the Monty Hall Problem is like this:

First of all, remember that Monty knows what’s behind every door. This is critical.

When you make your initial random choice from Doors A,B & C, there is a 2-in-3 chance that you will pick a goat. That is, two times out of every three your first random choice would give you a goat. Are we agreed on that point? Good. Therefore, on those two times out of every three, after Monty knows your choice, he will have no option but to open the door where the other goat is (presuming, of course, that he doesn’t want to show you the door with the car). Logically, therefore, Monty Hall allows you to know two thirds of the time where the car is† (that is, behind the door you didn’t choose). So you should always change your choice when he gives you the opportunity to do so.

It’s infuriatingly counter-intuitive. When I was first presented with the Monty Hall Problem I was convinced the choice was a mere 1/2 and therefore it made no difference if I changed or not. But the maths don’t lie. If the Word of the Cow isn’t good enough for you, go to the maths department at the University of California & San Diego and conduct yourself some practical trials. If you always change your choice when given the opportunity you will walk away with the car more often than not. You can also see the accumulated trials of everyone who has done the experiment before you: it’s inarguable – the best strategy is to swap doors when Monty gives you the choice!

Why do we have such difficulty with the Monty Hall Problem? I think the answer is twofold – firstly our brains are not naturally great at interpreting statistics, and secondly, The Monty Hall Problem is not strictly a problem of maths.

Statistically we can all see quite clearly that the chance of choosing the car initially is 1-in-3. We then tend to think that by being shown an ‘irrelevant’ door and given two remaining options there is an equal chance that either may hide the prize. This is in fact true; in a strict statistical sense, taken in isolation, the prize may indeed be behind either remaining door. But Monty (unwittingly, we must suppose) is not giving you that kind of choice. He is instead giving you the opportunity to change your mind about your first choice which is an entirely different thing altogether. And that opportunity is informed by the fact that Monty knows something about what’s behind the doors that you don’t.

In other words, a purely statistical experiment is muddied up by the fact that the experimenter knows something about the outcome and stirs that into the experiment, irrevocably removing the random element.

Or, put another way, if Monty doesn’t know what’s behind each of the doors (or, alternately, if you don’t tell Monty which door you’ve initially chosen) then the Monty Hall Show plays out exactly as your intuition might suggest. (Of course, if Monty doesn’t know what’s behind the doors, in all possibility he may reveal the car when he opens a (necessarily) random door to show you what’s behind it, immediately increasing your odds of walking away with the prize to 100%).

The Monty Hall Problem is a good reminder of how easily it is for the human brain to be lead astray, and why our intuitive grasp of things is not a reliable indicator of the way they really are…

___________________________________________________________________________

†Of course, on the one-in-three times you choose the car on your first go, Monty can show you either of two doors with a goat, in which case your chance of getting the car if you swap doors is merely 50/50. But that’s only for one out of every three times you randomly choose correctly on the first pick!

Correction courtesy of din.

___________________________________________________________________________

A couple of days back I was reading an article about statistical method in experiments in primate behaviour and the writer mentioned The Monty Hall Problem as a possible source of unintentional introduced error or experimenter bias.

Now The Monty Hall Problem is a fascinating mathematical conundrum, and since I know those kinds of things are always of interest to Cow Readers, I thought those of you who are not familiar with this puzzle might like to exercise your mental muscles on it.

The Monty Hall Problem goes like this:

You are on a game show with your host Monty Hall who is offering you the chance to walk away with the Car of Your Dreams. He shows you three doors, A, B & C.

“The Car of Your Dreams is behind one of these doors,” he says, “The other two doors each conceal a goat. As your Games Master, only I know which door conceals which object. Now, please choose a door to claim your prize!’

You choose your door. You tell Monty “I have chosen Door B!”

“Well done!” he says. “I knew you were a contestant of superior ability! But before we open your door, I’m going to open one of the other doors and show you what’s behind it.” He opens Door C to reveal a goat. “Now that you’ve seen what’s behind Door C,” he says, “I’m going to give you a special opportunity to stick with your chosen door, Door B, or change your choice to the other remaining door, Door A. I’ll give you ten seconds to have a think about it!”

Here’s the question: To win the car, is there any advantage in changing your mind and swapping from your initial choice of Door B to Door A?

Answers on my desk by end of class.

Popular Mechanics website recently carried an article about the ‘fragility’ of the nascent robotics industry and the unlikeliness that we’ll be seeing robots making our martinis anytime soon. Colin Angle, the CEO of iRobot (a company that specializes in ‘home robotics’*) said in his keynote address at the RoboBusiness conference in Pittsburgh last month that ‘the killer app that will drive the industry hasn’t yet emerged‘.

When he says ‘killer app’ I don’t think he’s talking about the heavily armed SWORDS† robots that the US military deployed in Iraq in 2007 and then immediately undeployed when the robotic gun ‘started moving when it was not intended to move‘… Before it could shoot when it was not intended to shoot, one speculates.

You all know my thoughts on robots. I’m thinking that we still have a ways to go even with trusting them to dust the china before we start handing out the AK47s. Not that the US Military (nor indeed the voting majority of the democracy that is the United States of America) seems to require much in the way of actual intelligence (artificial or otherwise) in that respect.

___________________________________________________________________________

*When they say home robotics, they evidently mean vacuum cleaners at this point in time…

†Special Weapons Observation Reconnaissance Detection System. Well whaddya want? It’s the military – they’re not known for their literary acumen.

Image swiped from the unmatchably geek-cool Modern Mechanix. Go there now and marvel at the treasures.

___________________________________________________________________________

The Continuing Misfortunes of Simple Graphics Man ~

#28: The Bloodthirsty Box.

In which SGM tangles with the deadly operatives from IKEA† who are bent on stealing his sanity and will go to any lengths to put him in the way of mortal danger.

___________________________________________________________________________

†International Ko-operative* for Evil Agents

*C’mon! These dudes always substitute a ‘k’ for a ‘c’! I’ve seen Get Smart! OK, OK, so KAOS isn’t an acronym… well at least you’ve got to give me that IKEA is evil.

Big thanks to Radioactive Jam for this latest installment in the saga of SGM!

___________________________________________________________________________